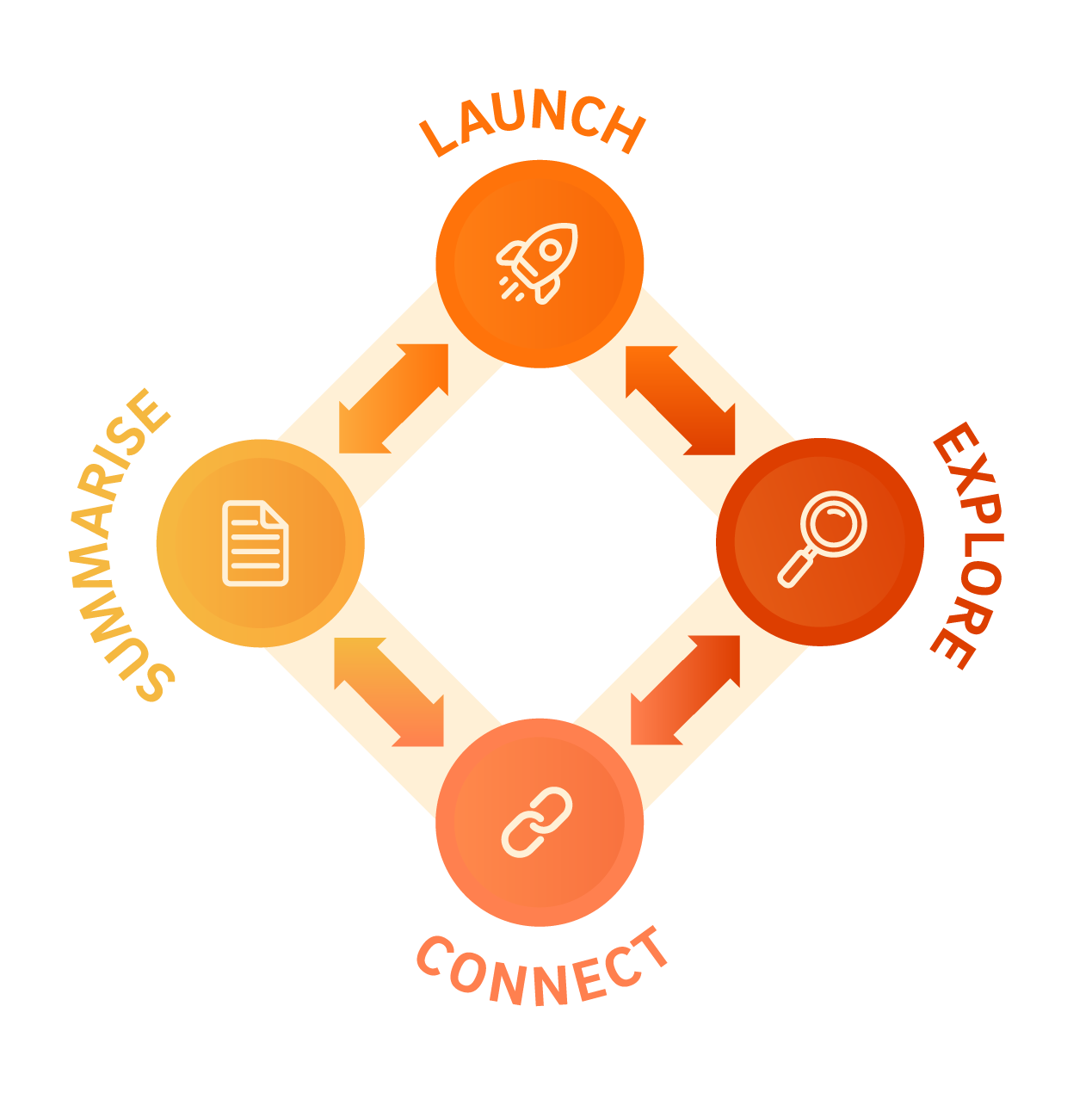
In reSolve, we use a four-phase instructional model for our problem-solving tasks: Launch–Explore–Connect–Summarise. We use this model as it enables students to engage in genuine mathematical inquiry as they explore open mathematical problems, make and test conjectures, form generalisations and justify their solutions. The teacher also plays a critical role in this lesson structure as they actively guide students’ mathematical inquiry.
Launch
The problem is introduced to the students by the teacher. A ‘hook’ or entry point, such as a realistic or imagined context or a puzzling problem, is often used as a tool to launch the problem. This hook provides a way for students to think and talk about the mathematics as they engage in the Explore phase. It is important that the teacher does not explain to students how to solve the problem in this phase. This allows students to access and build on their prior knowledge as they learn new content.
Explore
Through the Explore phase, students investigate the task and, as they do, they make sense of the important mathematical ideas addressed in the task. The teacher’s role is to notice and provoke student thinking. You do this through your observations of what they are doing and saying, and by engaging in conversations with students about the mathematics. Through your conversations, you ask carefully crafted questions to assess student understanding and to advance them towards the learning goal of the task or lesson. However, you do not provide students with a solution strategy, rather you guide and shape students’ thinking as they engage in mathematical sense-making.
Connect
This is the most important phase! This phase is about making connections: connections between the different strategies used by students and connections to the mathematical learning goal of the lesson.
The teacher strategically sequences some selected student strategies as the basis for a class discussion. These strategies are shared with the class and discussed so students can compare and highlight differences and similarities between the solution strategies. This phase is not a show and tell of different strategies. During the Connect phase, the teacher also explicitly addresses misconceptions or gaps in students’ understanding, teaches new mathematical ideas, and models formal ways of representing mathematics.
The Connect phase is also an important part of the Japanese lesson structure. In the Japanese lesson structure, the Connect phase is known as neriage which means ‘kneading up’ or ‘polishing up’. In the neriage phase, the teacher uses a whole-class discussion to knead together students’ strategies and ideas and then polish them to create a connected mathematical story. Neriage is regarded as the heart of the lesson, which can only happen because of the student problem-solving done at the beginning.
Summarise
This concludes the Connect phase, and it is where students briefly review the strategies that have been presented and discussed. The teacher speaks to the whole class to highlight and summarise the key ideas of the lesson and what students have learned during the lesson.
Build activities
In our problem-solving sequences, you will notice build activities. These build activities provide students with time and opportunity to practice their newly acquired skills and knowledge. Practicing in mathematics should be mindful for students, not mindless! For this reason, our build activities are based on:
- games that engage students in meaningful mathematical activity.
- puzzles that require students to think about mathematical ideas.
- sequences of number problems that are carefully crafted to highlight number relationships and build strategies.
Discuss with your colleagues:
- How is the Launch–Explore–Connect–Summarise model similar to and different from other instructional models that you have used in your mathematics teaching?
References
Clarke, D. (2009). Mind your language: Speaking in and about the mathematics classroom. In MAV 2009 Mathematics of Prime Importance: Proceedings of the Mathematical Association of Victoria 46th Annual Conference (pp. 34-49). Melbourne, Australia: The Mathematical Association of Victoria.
Mok, I. A. C. (2015). Research on mathematics classroom practice: An international perspective. In Selected regular lectures from the 12th International Congress on Mathematical Education (pp. 589-605). Springer International Publishing.
Walker, N. 2014. “Improving the effectiveness of the whole class discussion in the summary phase of mathematics lessons.” In J. Anderson, M. Cavanagh & A. Prescott (Eds.). Curriculum in focus: Research guided practice: Proceedings of the 37th annual conference of the Mathematics Education Research Group of Australasia (pp. 629–636). Sydney, Australia. MERGA.