Position determines place value 

There are 10 digits in our number system: 0, 1, 2, 3, 4, 5, 6, 7, 8 and 9. Using these digits, we are able to write an infinite range of numbers.

The position of a digit in a number gives it its unit value, while the digit itself represents the number of units in that position. We calculate the place value of a digit by multiplying the digit by its positional value.

Consider the two numbers 538 and 657 as examples. The 5 is worth 5 units in both numbers. However, the value of those units differs in each number. To find the place value of the digit 5 in 538 we multiply 5 by one hundred to establish that its place value is 5 hundred, or 500. To find the place value of the digit 5 in 657 we multiply 5 by ten to establish that its place value is 5 tens, or 50.

In place value systems, position matters!

The positional value of a digit is increased by a power of 10 with each place moved to the left, and the value decreases by a power of 10 with each place moved to the right. Therefore, a digit’s value increases or decreases by a power of 10 when it moves to a place which is either side of its current position.

The digit 0 is significant in our number system—it holds value! Take for example the number 204. It is not uncommon for a number like this to be described as 2 hundreds, no tens, and 4 ones. However, 204 has 2 hundreds, and those 2 hundreds are made up of 20 tens. There are just no tens remaining after they have all been grouped to make hundreds. As we talk about zero, it is important to stress that zero does hold value in a number.

Foundation

In Foundation, students develop fluency with the ten digits that comprise our number system. They learn how to use these digits to represent numbers and recognise the repeating pattern of the digits as they represent numbers beyond 9. Students learn about the structure of 10 and recognise the ‘teen’ numbers in relation to 10. At this point in time 2-digit numbers like 25 are typically perceived as a collection of 25 ones, rather than 2 tens and 5 ones.

Year 1

In Year 1 students encounter the powerful idea that position determines place value as they link the arrangement of tens and ones when counting collections to the total number for the collection. They start to associate the position of each digit with the unit it represents. By describing the size of the unit (tens or ones) and how many units are represented, students build the foundation for the understanding that the place value of each digit is found by multiplying the digit by the name of its position.

Students recognise that both the size of the unit and number of units are needed to describe the quantity represented. They recognise that the digit 2 in 12 represents a different value to the 2 in 21 because the 2s are in different positions, and that the 2 in 21 represents a value of 20 because it represents 2 groups of 10.

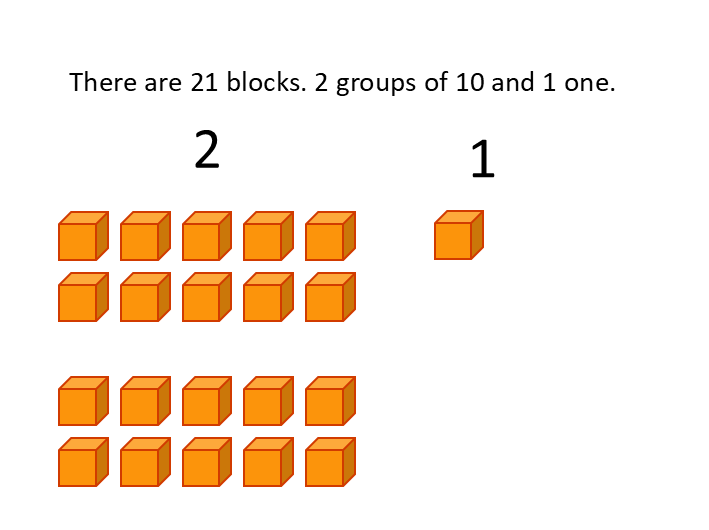
Year 2

In Year 2 students re-encounter position determines place value as they work with units in three different sizes, connecting proportional units of ones, tens and hundreds to their positions. They apply the powerful idea that position determines place value to name the positional value and the place value of digits in numbers to 1 000. They recognise that the place value of a digit is given by multiplying the digit by its positional value. Students learn to identify which digit is in the hundreds, tens or ones position, name which position a given digit is in, and state the place value of a given digit.

Year 3

In Year 3, the challenge of representing larger numbers highlights the need to generalise the positional and multiplicative properties so that they can be applied to interpret numbers that are more difficult to represent with proportional physical materials. When viewing a number like 475 512 students recognise that:

• the two digit 5s in the number do not represent the same value because they are in different positions.
• the place value of each digit is found by multiplying the digit by the name of its position. For example, the digit 7 represents a value of 70 000 because $7 \times 10 000$ is 70 000.

Year 4

In Year 4, students are introduced to the decimal point. They learn that the purpose of the decimal point in a number is to show where the ones position is when recording decimals. It is essential for students to understand that the decimal point is always in the same position, between the ones and the tenths, to avoid the misconception that the decimal point moves.

Students capitalise on the powerful idea that position determines place value as they learn to read and interpret decimals with up to 2 decimal places. They recognise that as the position of a digit determines its value, 0.5 and 0.50 represent the same value while 5.0, 0.5 and 0.05 represent different values. They know that they can determine the place value of any digit by multiplying the digit by the value of its position. Therefore, the place value of the digit 5 in 0.5 is $\frac{5}{10}$ or 5 tenths while the place value of the digit 5 in 0.05 is $\frac{5}{100}$ or 5 hundredths.

Year 5

In Year 5, students apply the idea that position determines value to state the place value of digits in decimals with up to 3 decimal places. They use the idea that position gives place value to compare and order decimals, record them on place value charts and position them on number lines in relation to other numbers.

Year 6

In Year 6 students apply their understanding of position gives place value to work with integers and decimals. They learn that numbers have both size and direction in relation to zero and that the direction affects its position on the number line but does not affect its size. As negative numbers are introduced, students need to use increasingly precise language. For example, 999 999 999 and -999 999 999 are equal in size they are located in opposite directions from zero. By comparison, 0.001 is a very small number, but it is higher than -999 000 000.

Year 7

In Year 7, students continue to apply the idea that position give place value as they round decimals to a given accuracy appropriate to the context and use appropriate rounding and estimation to check the reasonableness of solutions.

Year 8

In Year 8, students further extend their understanding of the powerful idea that position gives place value as they are introduced to irrational numbers and terminating and recurring decimals. They recognise that the real number system includes irrational numbers which can only be approximately located on the real number line. For example, they understand that the value of $ \pi $ lies somewhere between 3.141 and 3.142.

Year 9

In Year 9, students investigate the real number system more deeply by representing relationships between irrational numbers, rational numbers, integers and natural numbers. They identify the difference between exact representations and approximate decimal representations of irrational numbers.

Year 10

In Year 10, students learn to recognise the effect of using approximations of real numbers in repeated calculations and compare the results when using exact representations.