Statistics: Loopy aeroplanes
View Sequence overviewIntroducing new variables into an investigation means that we need to collect more data.
Whole class
Loopy aeroplanes PowerPoint
Each group
Equipment to make loopy aeroplanes:
- paper straws
- coloured paper
- scissors
- tape
- ruler
Each student
New loopy aeroplanes Student sheet
Lesson
Revise: We are investigating what is the best design for a loopy aeroplane. What have we learnt so far about different designs?
Discuss with students what they have learnt so far in the investigation.
Discuss: What are some other possible design modifications that we could investigate to determine what is “best”?
Allow students to offer different ideas about how the loopy aeroplane could be modified. Some modifications might include:
- using a medium sized loop with a large or small loop.
- using two loops the same size—two small loops, two medium loops, or two large loops.
- changing the width of the loops to create wide loops, normal loops and narrow loops.
- adjusting the placement of the loops, for example one loop at the front and one loop half-way along the straw.
- using a different length straw.
- using different lighter or heavier paper/card to make the loops.
Discuss with students how testing all of these different modifications could require hundreds of experiments, and as a class agree on only two modifications to investigate. We use loop size and width as the two selected modifications in these lesson notes.
Explain to students that the class will focus on two modifications:
- The size of the loops—small, medium or large
- The width of the loops—narrow, normal, or wide loops
Explain to students that both loops on their planes will be the same this time. This allows students to ensure all designs can be tested by the class.
As a class work out the different plane designs that need to be made. A tree diagram is a helpful way to show all the different loops for planes that need to be tested.
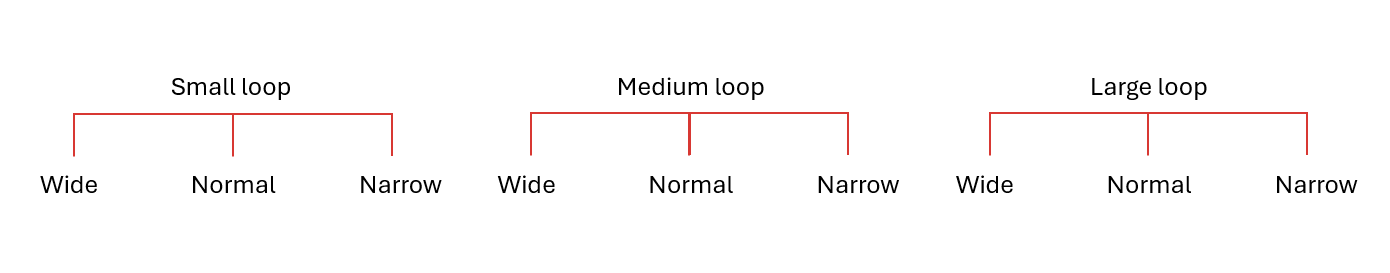
Planes galore!

Students are asked to think about possible design modifications that they might make as they consider, What loopy aeroplane design is best? Two modifications are chosen, the size of the loops and the width of the loops. There are three variations for each modification:
- The size of the loops—small, medium or large
- The width of the loops—narrow, normal, or wide loops
The students are then told that the loops on their plane must be the same this time, despite the fact they used two different loops on their first planes. Why did we make the decision to limit students plane designs in this way?
To conduct a fair test and gather reliable data, the students need to make and test all possible designs with these modifications.
If the two loops on each plane are the same, then there are just 9 different planes to make and test. This is very manageable for the class. If the two loops on the plane were allowed to be different then there are a lot more.
Let’s think through it…
There are 3 options for the size, three options for the width, and then these options can be repeated for the back loop. This means that there are (3 x 3) x (3 x 3) = 81, or 34 = 81, possible aeroplane designs that could be tested.
How many aeroplanes would the students need to make?
We know that one plane can be used to test up to two different designs by turning the plane around (as in the first activity). A plane with two different loops can be used to test two different designs. To calculate the number of planes that would need to be made:
| There are 81 different possible plane designs to be tested. | $9 \times 9 = 81$ |
| Of these planes, 9 have an identical loop at the front and back (e.g. a narrow small loop at the front and back). These planes can only be used to test one design. | $9 + (8 \times 9) = 81$ |
| The remaining planes can each be used to test two different designs, by turning the plane around. There are 72 remaining plane designs, which means that 36 different planes can be made. | $(8 \times 9)\div 2 = 36$ |
| Add together the first 9 planes and the second 36 planes. This gives 45 unique designs that can be made. | $9 + 36 = 45$ |
This means that there are 45 unique planes that would need to be made and 81 different plane designs to be tested! It would take a lot of time to make and test all these planes. It is better to limit the number of planes to be tested.
Students are asked to think about possible design modifications that they might make as they consider, What loopy aeroplane design is best? Two modifications are chosen, the size of the loops and the width of the loops. There are three variations for each modification:
- The size of the loops—small, medium or large
- The width of the loops—narrow, normal, or wide loops
The students are then told that the loops on their plane must be the same this time, despite the fact they used two different loops on their first planes. Why did we make the decision to limit students plane designs in this way?
To conduct a fair test and gather reliable data, the students need to make and test all possible designs with these modifications.
If the two loops on each plane are the same, then there are just 9 different planes to make and test. This is very manageable for the class. If the two loops on the plane were allowed to be different then there are a lot more.
Let’s think through it…
There are 3 options for the size, three options for the width, and then these options can be repeated for the back loop. This means that there are (3 x 3) x (3 x 3) = 81, or 34 = 81, possible aeroplane designs that could be tested.
How many aeroplanes would the students need to make?
We know that one plane can be used to test up to two different designs by turning the plane around (as in the first activity). A plane with two different loops can be used to test two different designs. To calculate the number of planes that would need to be made:
| There are 81 different possible plane designs to be tested. | $9 \times 9 = 81$ |
| Of these planes, 9 have an identical loop at the front and back (e.g. a narrow small loop at the front and back). These planes can only be used to test one design. | $9 + (8 \times 9) = 81$ |
| The remaining planes can each be used to test two different designs, by turning the plane around. There are 72 remaining plane designs, which means that 36 different planes can be made. | $(8 \times 9)\div 2 = 36$ |
| Add together the first 9 planes and the second 36 planes. This gives 45 unique designs that can be made. | $9 + 36 = 45$ |
This means that there are 45 unique planes that would need to be made and 81 different plane designs to be tested! It would take a lot of time to make and test all these planes. It is better to limit the number of planes to be tested.
Revisit the class protocols for making planes on slide 11 of Loopy aeroplanes PowerPoint. You will need to add protocols for the new designs that you are testing. For example:
| Length of card | Width of card |
Small loop – 15cm Medium loop – 20cm Large loop – 30cm | Narrow loop – 3cm Normal loop – 5cm Wide loop – 10cm |
Allocate each student a plane design to make and test. Ensure that each of the nine possible designs are being made by at least one student.
Provide students with New loopy aeroplanes Student sheet. Ask students to work in their small groups to plan their investigation. This sheet provides the following list of prompts to help groups plan their investigation:
- What planes will we make?
- What data do we need to collect?
- How will we record our data?
When they have developed their plan, provide the students with the necessary equipment and allow them time to make their new planes.