Multiplication: Trays of arrays
View Sequence overviewAn array can be partitioned to form smaller arrays. Adding the products of the smaller arrays gives the total in the original array.
Whole class
Trays of Arrays PowerPoint
Each group
Cupcake array picture
Blank A3 paper
Each student
Zachary and Maddie’s strategies Student sheet
Isabella and Archie’s Student sheet
Post-it notes for the gallery walk
Task
Use Trays of arrays PowerPoint to introduce the context of the reSolve Bakery. Show students the illustration on slide 4.
Discuss: What multiplication do you see? Some examples of multiplication represented in the picture include the arrays of cupcakes and bread rolls in the cabinet, and the bags of bread rolls sitting on the counter.
Show the picture of the cupcake array on slide 5.
Each day, six different flavours of cupcakes are made in the reSolve Bakery.
Pose the task: How many cupcakes are there altogether?
Choosing numbers and the design of the array
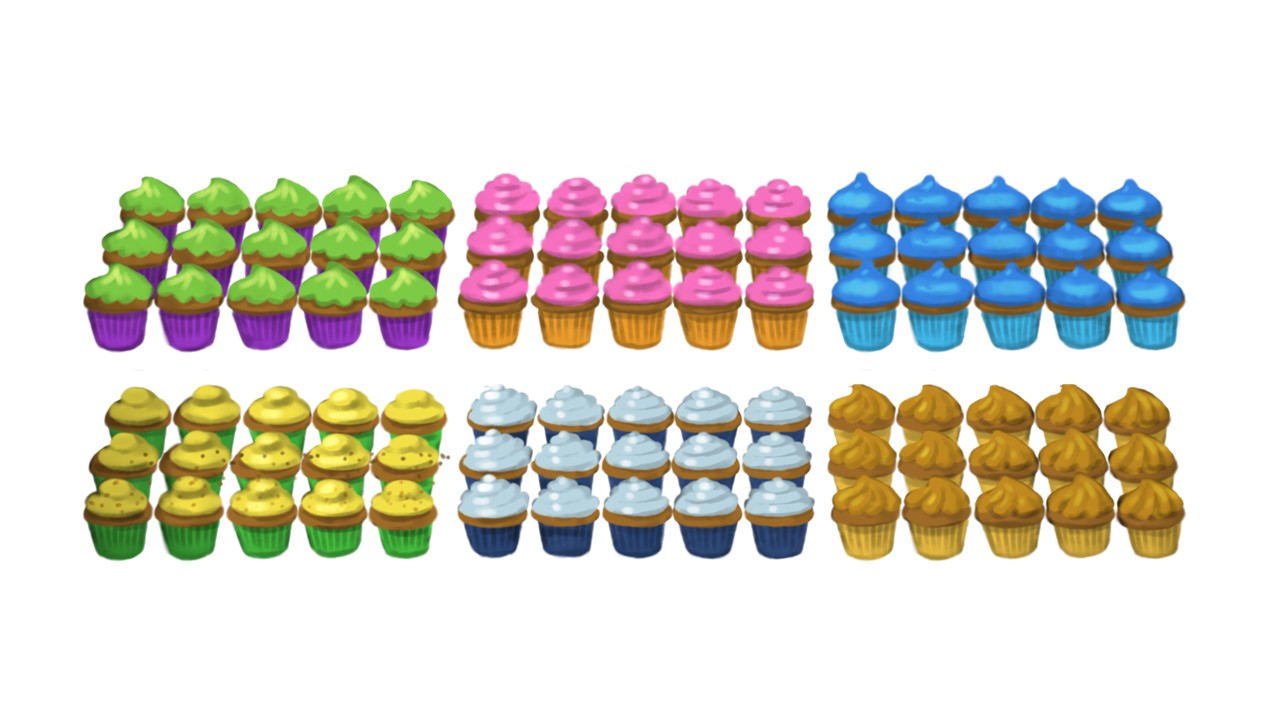
The array used in this question has been carefully designed and the numbers purposefully chosen.
Why has the array been designed this way?
6 x 15 can be seen in two different ways which lead to mathematically similar solutions.
- There are 6 smaller arrays of 15 cakes. These smaller arrays can easily be partitioned into a group of 10 and a group of 5. Partitioning the smaller arrays in this way creates 6 groups of 10 and 6 groups of 5, or (6 x 10) + (6 x 5).
- The larger array of cakes is arranged in 6 rows of 15. This larger array can be partitioned into a 6 x 10 array and a 6 x 5 array, which can also be solved using (6 x 15) + (6 x 5).
Why were the numbers 15 and 6 chosen?
15 was selected as it is typically an easier number for students to work with. Students can add together the arrays of 15 cakes across the rows or down the columns to find the total in the collection. 15 can also be arranged as a 5 x 3 array, which can then be partitioned into a 2 x 5 array to make 10 and a 1 x 5 array to make 5.
As a multiple of 2, doubling strategies can be used to multiply by 6. Students can calculate 3 x 15 using multiplication or repeated addition. They then just need to double this answer to find out 6 x 15.
The array used in this question has been carefully designed and the numbers purposefully chosen.
Why has the array been designed this way?
6 x 15 can be seen in two different ways which lead to mathematically similar solutions.
- There are 6 smaller arrays of 15 cakes. These smaller arrays can easily be partitioned into a group of 10 and a group of 5. Partitioning the smaller arrays in this way creates 6 groups of 10 and 6 groups of 5, or (6 x 10) + (6 x 5).
- The larger array of cakes is arranged in 6 rows of 15. This larger array can be partitioned into a 6 x 10 array and a 6 x 5 array, which can also be solved using (6 x 15) + (6 x 5).
Why were the numbers 15 and 6 chosen?
15 was selected as it is typically an easier number for students to work with. Students can add together the arrays of 15 cakes across the rows or down the columns to find the total in the collection. 15 can also be arranged as a 5 x 3 array, which can then be partitioned into a 2 x 5 array to make 10 and a 1 x 5 array to make 5.
As a multiple of 2, doubling strategies can be used to multiply by 6. Students can calculate 3 x 15 using multiplication or repeated addition. They then just need to double this answer to find out 6 x 15.
Ask students to work in pairs to solve the problem. Provide each pair with Cupcake array picture and a sheet of A3 paper. Ask students to use the A3 paper to create a poster of their solution method.
This task serves as a helpful pre-assessment task. The strategies that students use indicate their existing understandings of multiplication. Pose questions or prompts that help you to make sense of student thinking, for example:
- Explain your strategy to me.
- Why have you partitioned the numbers in that way?
- You have created smaller arrays from the larger array. Will the total number of cakes in all the smaller arrays be the same as the total number of cakes in the large array? How do you know?
Students will use a range of strategies to determine the total number of cupcakes. The purpose at this early stage in the sequence is not to point students to using a particular strategy but rather to take note of the strategy and what this indicates about students’ thinking and understanding.
Consider:
- Do students use additive or multiplicative thinking to solve the problem?
- Do students use of strategies demonstrate an understanding of the multiplicative properties of associativity or distributivity?
Watch the Distributive and associative properties of multiplication professional learning video embedded in this step to learn more about strategies that use these properties.
Student work samples
Look at these work samples and see how these students have solved the problem.
Discuss with colleagues: What do these students know and what have they shown that they can do? Give evidence from the work samples for your statements.
Look at these work samples and see how these students have solved the problem.
Discuss with colleagues: What do these students know and what have they shown that they can do? Give evidence from the work samples for your statements.
The importance of mathematical talk
We have suggested working in pairs or small groups during the Explore phase of this task to promote mathematical discourse. Mathematical talk in the classroom is fundamental to both knowing and doing mathematics. Students should have regular opportunities to work on and talk about solving problems in community with peers. It is likely that each student in the group will approach the task in slightly different ways. Through discourse, students put forth claims and justify them as well as listening to and critiquing claims of others. Solving problems in community provides a venue for more talking and listening than is available when working individually or in a teacher-led lesson.
We have suggested working in pairs or small groups during the Explore phase of this task to promote mathematical discourse. Mathematical talk in the classroom is fundamental to both knowing and doing mathematics. Students should have regular opportunities to work on and talk about solving problems in community with peers. It is likely that each student in the group will approach the task in slightly different ways. Through discourse, students put forth claims and justify them as well as listening to and critiquing claims of others. Solving problems in community provides a venue for more talking and listening than is available when working individually or in a teacher-led lesson.
Display students’ work around the classroom in preparation for a gallery walk.
Review the original task that students were asked to solve and ask students to think about what they expect to see as they complete the gallery walk.
Ask students to consider the following questions as they look at others’ work:
- Look at how other students have solved the problems. What do you notice?
- Which strategies were particularly helpful for working out the total number of cupcakes? Why?
Conduct the class gallery walk.
At the end of the class gallery walk, allow students time to read and reflect on any post-it notes left on their work. They may choose to adjust or change to their solution strategies and/or recording methods.
Gallery walk
In a gallery walk the role of the student is to critically view and review others’ mathematical activity. They need to think more broadly than the strategy they have personally used, as they consider how their thinking fits with the representations of thinking used by others in the class.
In this gallery walk, students are asked to look at how others have solved the problem and consider which strategy/strategies are the most helpful when determining the total number of cakes in the array. As students look at others’ strategies, they are able to reflect on and refine their own approach to solving the problem.
In a gallery walk the role of the student is to critically view and review others’ mathematical activity. They need to think more broadly than the strategy they have personally used, as they consider how their thinking fits with the representations of thinking used by others in the class.
In this gallery walk, students are asked to look at how others have solved the problem and consider which strategy/strategies are the most helpful when determining the total number of cakes in the array. As students look at others’ strategies, they are able to reflect on and refine their own approach to solving the problem.
What is a gallery walk?
In a gallery walk, students move around the classroom like they are in an art gallery, in silence or whispering with a partner. Students use post-it notes to post comments and questions about the mathematics they see. They should be encouraged to take their time to respectfully read and respond to the work of others.
At the end of the gallery walk students should be given time to read and reflect on the post-it notes that have been left for them. Students should be allowed time to modify their work if they would like to. The questions and feedback on these notes will help refine students’ thinking and the manner of their mathematical recording. It is also likely that the students will have developed new thinking as they critically viewed and reflected on others’ work, and it is important that students have the opportunity to act on this new learning.
In a gallery walk, students move around the classroom like they are in an art gallery, in silence or whispering with a partner. Students use post-it notes to post comments and questions about the mathematics they see. They should be encouraged to take their time to respectfully read and respond to the work of others.
At the end of the gallery walk students should be given time to read and reflect on the post-it notes that have been left for them. Students should be allowed time to modify their work if they would like to. The questions and feedback on these notes will help refine students’ thinking and the manner of their mathematical recording. It is also likely that the students will have developed new thinking as they critically viewed and reflected on others’ work, and it is important that students have the opportunity to act on this new learning.
Provide students with Zachary and Maddie’s strategies Student sheet. Explain that these two students solved the problem in different yet similar ways.
Ask: How are these strategies similar and how are they different?
Allow students time to explore the similarities and differences. Have students record their noticings on their student sheet.
Provide students with Isabella and Archie’s strategies Student sheet. Explain that these two students also solved the problem in different yet similar ways.
Ask: How are these strategies similar and how are they different?
Allow students time to explore the similarities and differences. Have students record their noticings on their student sheet.
Comparing strategies
Transcript not yet available
This sequence is designed to build students’ understanding of the distributive and associative properties of multiplication and how these properties can be applied to solve multiplication problems. In this video, we illustrate how the distributive and associative properties of multiplication are linked to the four strategies that students compare in the Connect phase of this task.
Similarities and differences
Students are shown four different strategies in this Connect phase, and they discuss the similarities and differences that they notice. The key similarity that we want students to notice is that the array can be partitioned into smaller parts to aid computation. It is important that students see that the array must be partitioned fully, no parts can be left out. The total is then calculated by adding together the products of the smaller arrays.
Students are shown four different strategies in this Connect phase, and they discuss the similarities and differences that they notice. The key similarity that we want students to notice is that the array can be partitioned into smaller parts to aid computation. It is important that students see that the array must be partitioned fully, no parts can be left out. The total is then calculated by adding together the products of the smaller arrays.
Use the Trays of arrays PowerPoint to support the discussion. Share Zachary and Maddie’s strategy on slide 6.
Discuss:
- How are these strategies different?
- Zachary doubles 15 and Maddie adds 15 three times.
- How are these strategies similar?
- Both strategies partition the array into groups of 15 and these groups of 15 are then added together.
Share Isabella and Archie’s strategy on slide 7.
Discuss:
- How are these strategies different?
- Isabella partitions each small cupcake array of 15 into a group of 10 and a group of 5, making 6 groups of 10 and 6 groups of 5.
- Archie partitions the full cupcake array into a 6 x 10 array and a 5 x 6 array.
- How are these strategies similar?
- Both strategies can be solved using the expression (6 x 10) + (6 x 5).
Look at Strategies 1-4 as a group on slide 8.
Discuss:
- What is similar about each of these strategies?
- In each case, the larger array has been fully partitioned to form smaller arrays based on known multiplication facts. All the partial products formed are then added together to find the total in the whole collection.
Whole class discussion
The purpose of whole class discussion is to make the mathematics visible, so that students can develop their thinking beyond what they are able to do on their own or in small groups. Through discussions, teachers can build a thoughtful community of mathematical thinkers, where students actively participate in sharing their ideas and listening to those of others.
In making the mathematics visible, you guide students in noticing and building connections between mathematical concepts that they may have seen as disconnected. It is through this deeper understanding of the mathematics that students can begin to form generalisations.
The purpose of whole class discussion is to make the mathematics visible, so that students can develop their thinking beyond what they are able to do on their own or in small groups. Through discussions, teachers can build a thoughtful community of mathematical thinkers, where students actively participate in sharing their ideas and listening to those of others.
In making the mathematics visible, you guide students in noticing and building connections between mathematical concepts that they may have seen as disconnected. It is through this deeper understanding of the mathematics that students can begin to form generalisations.
Ask the students to consider whether the strategy that they used was most similar to Zachary, Maddie, Isabella, or Archie’s strategy. Discuss how in each instance, what was known was used to work out what was unknown.
Explain: We can calculate the total number of objects in an array by partitioning the large array into smaller arrays. The total number of objects in the smaller arrays are then added together to find the total number of objects in the large array.
As a class, look at some of the ways that students partitioned the larger array to create smaller arrays using known multiplication facts.
Create a class display using the students' posters, using the summary statement from above as a title for the display. Read the Class display professional learning embedded in this step to learn how this display can be used to build a shared understanding amongst the students.
Class display
In the Summarise phase, the following statement is presented to students:
We can calculate the total number of objects in an array by partitioning the large array into smaller arrays. The total number of objects in the smaller arrays are then added together to find the total number of objects in the large array.
This statement reflects the learning goal for the task and is the understanding that we want all in the class to share. As the sequence progresses, this shared understanding is built on.
Create a banner or poster of this shared understanding and display the students’ posters of solutions strategies underneath or around the shared understanding. This provides the opportunity for students to revisit and reflect on this understanding and the ways that that it is reflected in the different solutions strategies used by students. The display is a way to help ensure the understanding is truly shared by all in the class.
Presenting everyone’s work with this statement communicates that everyone has contributed to and participated in developing this understanding as shared in the class, regardless of how complex or sophisticated their thinking may or may not be.
In the Summarise phase, the following statement is presented to students:
We can calculate the total number of objects in an array by partitioning the large array into smaller arrays. The total number of objects in the smaller arrays are then added together to find the total number of objects in the large array.
This statement reflects the learning goal for the task and is the understanding that we want all in the class to share. As the sequence progresses, this shared understanding is built on.
Create a banner or poster of this shared understanding and display the students’ posters of solutions strategies underneath or around the shared understanding. This provides the opportunity for students to revisit and reflect on this understanding and the ways that that it is reflected in the different solutions strategies used by students. The display is a way to help ensure the understanding is truly shared by all in the class.
Presenting everyone’s work with this statement communicates that everyone has contributed to and participated in developing this understanding as shared in the class, regardless of how complex or sophisticated their thinking may or may not be.