Multiplication: Trays of arrays
View Sequence overviewWhen multiplying two numbers, if we halve one number and double the other the total remains the same. Equivalence in multiplication is maintained no matter how the numbers are grouped.
Whole class
Trays of arrays PowerPoint
Each group
Blank A3 paper
Trays of bread rolls picture, printed on A4 and cut in half
Task
Use Trays of arrays PowerPoint to continue the context of the reSolve Bakery. Show students the illustration on slide 17.
Revise: We have learnt that we can solve a difficult multiplication problem by partitioning factors to create smaller problems. Partitioning factors to create well-known multiplication facts, such as multiples of ten, is particularly helpful. The products of these smaller problems are then added together to find the product of the original problem.
Show the picture of the baker and the bread rolls on slide 18.
The baker bakes five trays of bread rolls each day. There are a dozen bread rolls on each tray. The baker then packs all these rolls into bags. Each bag has exactly six bread rolls.
Show the picture of the second bread roll array on slide 19.
Pose the task: How many bags of six bread rolls will there be?
Provide each pair with a sheet of A3 paper and access to Trays of bread rolls picture to use if they choose.
Allow students time to investigate the problem and to present their solution as a poster on the A3 paper.
How do students divide the bread rolls into bags of six? Do students:
- Share by ones: Prompt students to think about how they could efficiently make groups of six.
- Create groups of six without using an array: Ask students:
- Are you confident that there are six in each array?
- How could you arrange the groups so that you know there are six at a glance?
- Partition the array of 12 into two arrays of six: Encourage students to think about how they might describe their strategy in words or record it as an equation.
Do students notice the relationship between the number of bread rolls on one tray and the number of bread rolls in one bag? Don’t draw their attention to this at this stage of the task—it will be the focus of the Connect phase. If students do comment on this relationship, ask them to consider why it exists.
Work samples
Look at how these students have solved the problem.
Discuss with colleagues: What do these students know and what have they shown that they can do? Give evidence from the pictures for your statements.
Look at how these students have solved the problem.
Discuss with colleagues: What do these students know and what have they shown that they can do? Give evidence from the pictures for your statements.
Invite some students to share their solutions with the class. Consider how the different strategies are different and how they are similar.
Record the solution to the problem on the board in the following way:
5 trays of 12
10 bags of 6
Discuss:
- What relationships do you notice?
- The number of bags is double the number of trays and the number of rolls in a bag are half the number of rolls that are on a tray.
- Why do these relationships exist?
- The bread rolls on one tray are packed into two bags. To do this, we halve the large array of 12 rolls on one tray into two smaller groups of six. This means we need twice the number of bags as trays.
Explain to students that we can make sense of this relationship using the array and numbers. Show students Trays of arrays PowerPoint slides 20 and 21. These slides illustrate how the associative property applies to this task.
Discuss how the array helps to make sense of these relationships and that equivalence in multiplication is maintained no matter how the numbers are grouped.
The associative property of multiplication
The associative property of multiplication states that the multiplication of a set of numbers gives the same output no matter how the numbers are grouped. So,
$$(a \times b) \times c = a \times (b \times c)$$
Let’s see how this property applies to this problem.
There are 5 trays of bread rolls with 12 on each tray.
$$5 \times 12$$
The 12 bread rolls on one tray can be halved into 2 groups of 6. This creates 5 trays of bread rolls with 2 groups of 6 on each tray.
$$5 \times (2 \times 6)$$
There are 5 trays, and each tray has enough bread rolls for 2 bags. There are 6 bread rolls in each bag.
$$(5 \times 2) \times 6$$
We halve the 12 to make 2 groups of 6 bread rolls on each tray, which means that there are double the number of bags as trays.
$$5 \times (2 \times 6) = (5 \times 2) \times 6$$
Halve the 12 Double the 5
The associative property of multiplication states that the multiplication of a set of numbers gives the same output no matter how the numbers are grouped. So,
$$(a \times b) \times c = a \times (b \times c)$$
Let’s see how this property applies to this problem.
There are 5 trays of bread rolls with 12 on each tray.
$$5 \times 12$$
The 12 bread rolls on one tray can be halved into 2 groups of 6. This creates 5 trays of bread rolls with 2 groups of 6 on each tray.
$$5 \times (2 \times 6)$$
There are 5 trays, and each tray has enough bread rolls for 2 bags. There are 6 bread rolls in each bag.
$$(5 \times 2) \times 6$$
We halve the 12 to make 2 groups of 6 bread rolls on each tray, which means that there are double the number of bags as trays.
$$5 \times (2 \times 6) = (5 \times 2) \times 6$$
Halve the 12 Double the 5
Noticing relationships
We want students to notice the relationship between the numbers in this problem. We define “noticing” as the active process of attending to and making sense of mathematical patterns and relationships.
We want students to see the relationships between the numbers in the problem and, very importantly, we want students to make sense of why these relationships exists. As they make sense of the relationships between the numbers they are working towards a generalised understanding of the associative property of multiplication.
We want students to notice the relationship between the numbers in this problem. We define “noticing” as the active process of attending to and making sense of mathematical patterns and relationships.
We want students to see the relationships between the numbers in the problem and, very importantly, we want students to make sense of why these relationships exists. As they make sense of the relationships between the numbers they are working towards a generalised understanding of the associative property of multiplication.
Use Trays of arrays PowerPoint slide 21 to review the learning from this task.
Review: To find out how many bags of bread rolls there will be, we halved 12 to make 2 groups of 6, which we represented as $2 \times 6$. We had 5 trays and there were enough rolls on each tray to pack 2 bags of 6 bread rolls. So, to find the number of bags, we multiplied 5 by 2 to make 10, which we represented as $5 \times 2$.
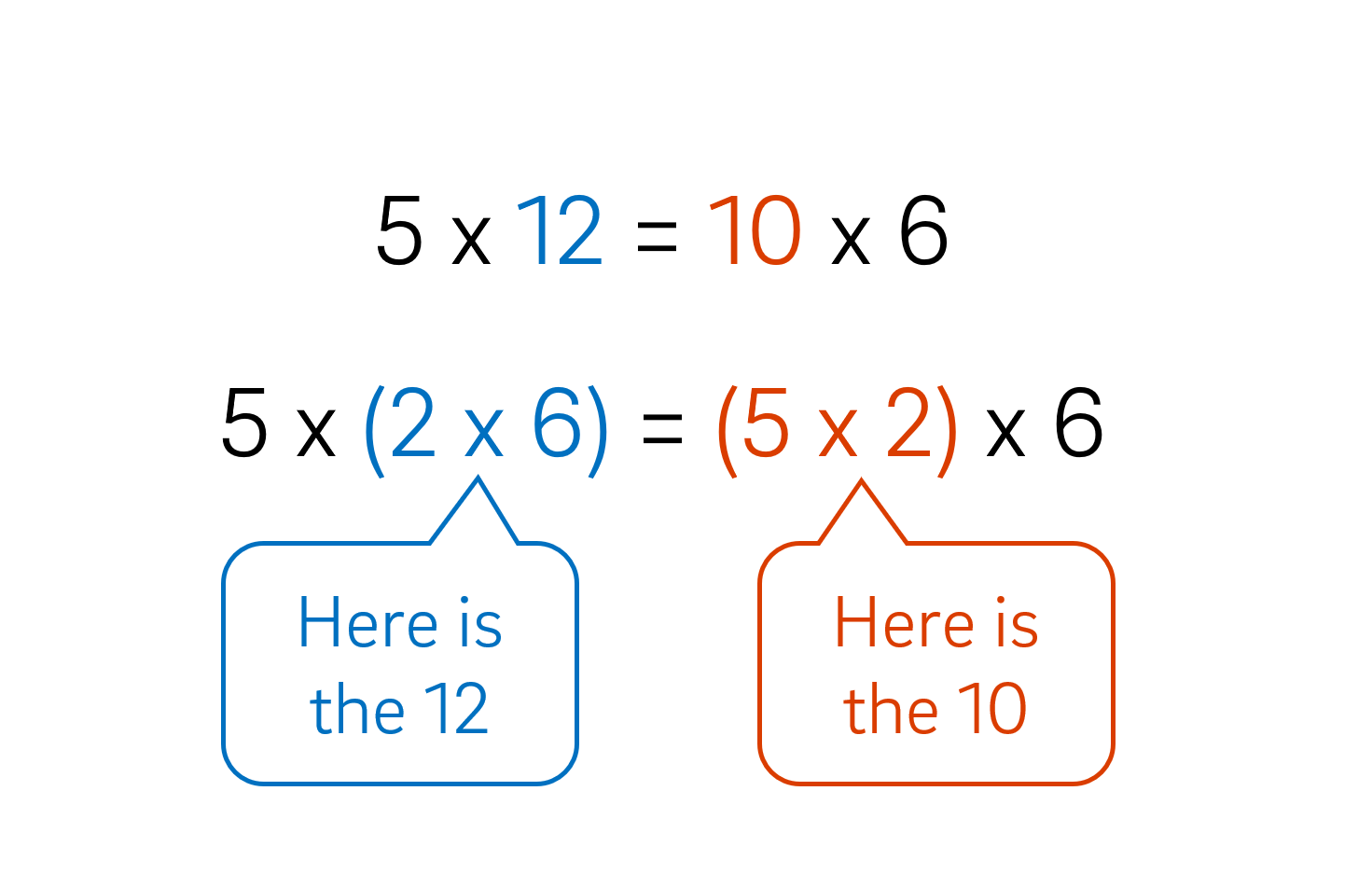
On one side of the equation the 2 was grouped with the 6 and on the other side the 2 was grouped with the 5.
Explain: Equivalence in multiplication is maintained no matter how the numbers are grouped. This helps us understand why halving one number in a multiplication means we need to double the other number to ensure the total remains the same.
Add to the class display using the students' posters from this task, using the summary statement from above as a title for the display. Read the Class display professional learning embedded in this step to learn how this display can be used to build a shared understanding amongst the students.
Shared understandings
The statement presented in this Summarise phase is the shared understanding that we want for all students in the class.
Extend the class display of work to include this Summarise statement and students’ work samples. This provides the opportunity for students to revisit and reflect on this understanding and the ways that that it is reflected in the different solutions strategies used by students. The display is a way to help ensure the understanding is truly shared by all in the class. Students can also see how their shared understanding is developing through the sequence.
Presenting everyone’s work with this statement communicates that everyone has contributed to and participated in developing this understanding as shared in the class, regardless of how complex or sophisticated their thinking may or may not be.
The statement presented in this Summarise phase is the shared understanding that we want for all students in the class.
Extend the class display of work to include this Summarise statement and students’ work samples. This provides the opportunity for students to revisit and reflect on this understanding and the ways that that it is reflected in the different solutions strategies used by students. The display is a way to help ensure the understanding is truly shared by all in the class. Students can also see how their shared understanding is developing through the sequence.
Presenting everyone’s work with this statement communicates that everyone has contributed to and participated in developing this understanding as shared in the class, regardless of how complex or sophisticated their thinking may or may not be.